You can draw concepts and I can interpret them.
Sort of like Death / Close Your Eyes Draw Quote
Sort of like Death / Close Your Eyes Draw Quote
-
Did you mean to say Mr. Fantastic?
Not inherently bad, but should generally be avoided. With ToT, I was basically just being lazy (incidentally, the "full" (non organya) version of ToT also has very little change of texture, but in that case it seems to fit well). Off the top of my head, some situations where it is not bad would be in a very short piece of music, or in game and film music.
:0 what are we thinking
I don't know exactly, but I'd say "Most". If it's truecolour all you need is the pixel data really..
Evernote is "onefreetea"
Nah, my goal was minimizing the filesize so I went as bare-bones with the header as I possibly could.
I think much of the bitmap file header is stuff to do with reverse-compatibility with older formats, and also making them faster to read (the pointers to the pixel data for instance)
or storing other information for I don't know what.
That's basically the format. A linear sequence of events corresponding to RLE-compressed pixel data, with bits per pixel optimized. Is that what you meant?Code:private String imgTag; //"NIM1"; 4E 49 4D 31 private short flags; /*------Flags----- * 0x1 - Horizontal type * 0x2 - Vertical type * 0x4 - TL-BR Diagonal type * 0x8 - BL-TR Diagonal type * 0x10 - 0x8000 Unused ---------------*/ private byte BPP; private byte transBits; private int nimWidth; private int nimHeight; //if BPP <= 8 Pallette size is 4* (2^BPP) private Palette palette; private int numEvents; private NimEvent[] eventData; // each event is 8 bits len + BPP bits colour data
I could even be something dumb like the first derivative of the second derivative of location with respect to time with respect to force. That would mean that my units would be 1/mass.
Tually, I still could be a third derivative of location, but just not with respect to time.
No.
No.
So I think my final verdict is that I'm not coming in October. When I asked Nox if he'd be interested in a summer meeting, he very wisely said that it depended largely on how successful the October one is. It would kinda suck if, after all this planning, it ended up being this lame thing where we're all like "Ok, we came, we saw each other, now what?". So y'all can let me know how the October one goes, and then we'll discuss whether or not it'd be worthwhile to have a summer one.
Also, I feel morally obligated to rephrase what I told you on IRC last night 'bout not using notes outside of scales. What I should have said was something like "Feel free to use those notes, but, for the time being, I'm not gonna give you any guidance on how to do so - I'm just letting you fend for yourself". Of course, you're probably smart enough to figure out that that was implied for yourself, but I still felt like kind of a third derivative for just telling you no.
edit: kudos if you can get my sig. I think it's a bit more difficult than yours.
jup
Okie dokie.
Lace said:
98% of the time, it is correct to assume that. Chords with 4 notes are usually called 7 chords, 5 notes = 9 chords, 6 notes = 11 chords, and 7 notes = 13 chords. The latter 3 are rarely seen outside of jazz. Extending that pattern in the opposite direction would have us believe that chords with 3 notes are called 5 chords, but this is not the case. Actually, the term "5 chord" refers to a chord with 2 notes - the root and the note a perfect 5th above the root. This is the same as a major or minor triad with the 3rd omitted.
Lace said:
Those 7 chords are called the diatonic triads of G minor. And now we get to the fun part of explaining why Ionian and Aeolian (major and minor) modes are the most widely used, Locrian is the least widely used, and the other 4 (Dorian, Phrygian, Lydian, and Mixolydian) are somewhere in between.
As you pointed out, the diatonic triads of G Aeolian are G min, A dim, Bb maj, C min, D min, Eb maj, and F maj. As it turns out, all Aeolian keys have diatonic triads that form the pattern: min, dim, maj, min, min, maj, maj.
You also mentioned seeing little numbers used to denote the chords. My guess is you're referring to the figured bass symbols. Roman numerals are used to indicate diatonic triads, (so the first is 1, the second is 2, etc.). Uppercase indicated major, lowercase indicates minor, uppercase with a plus sign indicates augmented, and lowercase with a degree sign indicates diminished. So the figured bass symbols used to indicate the diatonic triads of an Aeolian (minor) scale are: i, iiº, III, iv, v, VI, VII.
By taking this pattern and "rotating" it, we get the other 6 modes:
Ionian: I, ii, iii, IV, V, vi, viiº
Dorian, i, ii, III, IV, v, viº, VII
Phrygian: i, II, III, iv, vº, VI, vii
Lydian: I, II, iii, ivº, V, vi, vii
Mixolydian: I, ii, iiiº, IV, v, vi, VII
Aeolian: i, iiº, III, iv, v VI, VII
Locrian: iº, II, iii, iv, V, VI, vii
Also, each of the 7 diatonic triads is given a name based on its position:
1 - tonic
2 - supertonic
3 - mediant
4 - subdominant
5 - dominant
6 - submediant
7 - leading tone
The tonic is the most important, since it is the "home" triad, where everything should resolve to. Therefore, the quality of a mode is determined by the quality of its tonic triad. Ionian, Lydian, and Mixolydian are major modes, Dorian, Phrygian, and Aeolian are minor modes, and Locrian is a diminished mode.
The reason for the relative amount of use each mode gets stems from the placement of the tri-tone. You may recall me saying...
From a physics perspective, the reason this interval sounds so harsh and piercing is because of its frequency ratio. Our ears "like" to hear simultaneous frequencies that are in "nice" ratios (i.e. 1:2, 1:3, 2:3, 1:4, 3:4, etc.). A very simplistic way to say this is that nice ratios sound good and not-so-nice ratios (17:23, 8:19, 21:64, etc.) sound bad. I don't like to use the words "good" and "bad" in this scenario, however, because they are overly simplistic. A better way to describe it would be "consonant" and "dissonant", or, in more familiar terms, "stable" and "unstable".me said:
Because this phenomenon is based on the physical way our ears perceive sound, it is universal across all cultures and musical styles. What's not so universal is the way consonance and dissonance are used to create musical ideas. One of the most common paradigms (which we will be focusing on) is to have a sense of "tension and release" where more dissonant chords resolve to more consonant ones. Even within this paradigm, different styles differ significantly in what range of the consonance/dissonance spectrum they tend to use. To give a few examples:
Medieval/Renaissance: extreme consonance - moderate consonance
Baroque/Classical: moderate consonance - moderate dissonance
Romantic: moderate consonance - extreme dissonance
Jazz/Modern: moderate dissonance - extreme dissonance
As you can see, the general trend in Western music over the past several centuries has been one of ever increasing dissonance.
Some common intervals and their frequency ratios:
P8 - 1:2
P5 - 2:3
P4 - 3:4
M3 - 4:5
m3 - 5:6
M6 - 3:5
m6 - 5:8
M2 - 8:9
tri-tone - 1:sqrt(2)
This explains why the tri-tone is so dissonant - not only is it not a nice ratio, it's not even rational! It is worth noting, however, that, on a modern piano (or other keyboard instrument, as well as almost all electronic music systems like organya, pxtone, FLStudio, a digital piano, etc.), none of the intervals are rational (except the octave). The modern piano uses an "equal-temperament" tuning system, meaning every half-step is equal. In order to make the octave a 1:2 ratio, each half step must have a 1:2^(1/12) ratio. For all intervals except the tri-tone, the minor 2nd, and the major 7th, the irrational frequency ratio is so close to one of the nice ratios listed above, that most people's minds will subconsciously "round" the irrational interval to the nearest nice rational one, and the slight dissonance is not perceived. These three intervals therefore sound the most dissonant.
In Common Practice era music (recall from page 5 of this convo that this refers to Baroque, Classical, and Romantic period music), the tri-tone is used to create dissonance much more often than the minor 2nd and major 7th. The main reason for this is voice leading. We'll cover voice leading in greater detail later later, but for now I'll just say that voice leading is concerned with melody (the "horizontal" view of notes) while consonance/dissonance is concerned with harmony (the "vertical" view of notes). Common Practice era music tends to put a higher importance on voice leading than other styles do. The "proper" way to resolve a tri-tone (which usually gives the most satisfying voice leading) is to move one note down a half-step (sometimes two half-steps, but we won't worry about that for now) and the other up a half-step, so the tri-tone either expands to a minor 6th or contracts to a major 3rd.
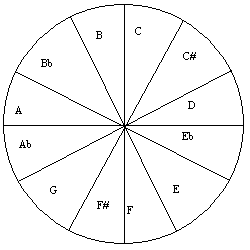
One way I like to think of it is in terms of the chromatic circle from page 6 of this convo:
Suppose I have two instruments playing a piece of music. I imagine the two instruments as being two small objects moving around the edge of the circle, connected by a spring. When the interval between them is a minor 2nd or major 7th, they're too close together, and the spring is compressed, which pushes them apart. When the interval between them is a tri-tone, they're on opposite sides of the circle, and the spring is stretched, which pulls them together.
All this crap was allegedly leading up to a description of why some modes are more commonly used than others, so here we go (finally).
Consider the C major scale (all white keys, starting on C). There are 7 notes in this scale, so there are 7*6/2=21 pairs of notes. As it turns out, only one of these 21 pairs forms a tri-tone, namely B & F. To resolve this tri-tone, and stay within the key of C major, we must move the B up to a C and the F down to an E (going the opposite direction wouldn't work, because we'd end up with Bb/A# and Gb/F#, neither of which are in the C major scale). Notice that both of these notes are in the tonic triad of C major (C, E, G). This is very convenient, because it means that the only tri-tone inherent in the C major scale naturally "wants" to resolve to the home triad of C major. And since all major scales have the same series of intervals and only differ by their starting note, this applies to all major scales, and not just C.
That explain why Ionian mode (major) works well, but what about the other 6 modes? Recall that all 7 modes have the same series of intervals, just in different "rotations". This means that all 7 modes have exactly one tri-tone which has a specific way it wants to resolve. For simplicity, let's just look at the scales that consist of all white keys (D Dorian, E Phrygian, F Lydian, G Mixolydian, A Aeolian, and B Locrian). These scales all have the same notes as C major, so the tri-tone will be B & F resolving to C & E. In A Aeolian (minor), like in C major, C & E are part of the tonic triad, so the tri-tone resolves to the home key. In B Locrian, B & F are part of the tonic triad, so the tri-tone actually resolves away from the home key. In the other four modes, neither B & F nor C & E are subsets of the tonic triad.
So, to summarize:
Ionian and Aeolian are convenient to compose in because the tri-tone resolves to the home key.
Locrian is inconvenient to compose in becase the tri-tone resolves away from the home key.
Dorian, Phrygian, Lydian, and Mixolydian are somewhere in between because the tri-tone resolution is unrelated to the home key.
Yup that's pretty much correct. The only thing that surprised me was the thing saying all triads have 6 inversions. That is one way of thinking of it, but usually the word "inversion" just describe which note of the chord is in the bass, so there are only n inversions of an n-part chord rather than n! In general, only the top and bottom notes have a significant impact on the sound of a chord, so you could also say that there are n^2 inversions of an n-part chord (remember the top and bottom note could be the same).
With the middle notes, the order doesn't matter so much as the quantity of each note, as well as the approximate statistical distribution of the notes. For example, a C major triad consisting of 3 Cs, 3 Es, and 3 Gs will sound significantly different from one with 1 C, 6 Es, and 2 Gs. Also, a chord whose notes are all fairly centrally located on the keyboard will sound very different from one whose notes are at the extremes of the keyboard, even if they're the same chord.
With the diminished triad, the note a minor 3rd above the minor 3rd is called the diminished 5th.
Was gonna write more but I gotta go to class now. Also,
me said:
Hopefully I'll be able to tell you those reasons very soon.
I am curious to see what comes of it
I tried to come up with a concept that was difficult but not impossible (homing) and then threw on a bunch of arbitrary specifications
My other idea was a spur weapon that could be fired at any angle, but I figured it would be pretty ineffective without mouse controls.
Possibly.. I don't think I've actually seen it in person :0
Moderating is serious business
Nobody is safe. Not even me.
Alright, so just to get an idea of how much you know, explain everything you know about chords.
it
^
|
|
way
|
|
<- head -
Loading…
-
Loading…
-
Loading…
-
Loading…
